HOW TO: count complex rhythms while playing guitar

Image 1 of 4

HOW TO: count complex rhythms while playing guitar

Every month, Guitar Techniques attempts to answer guitarists' playing posers and technical teasers with expert and practical advice. Here's one about complex - or awkward - rhythms and how to count them…

The question:

Dear GT,

Can you explain how to count awkward rhythms? I think I am okay on the basics - semibreves, minims, crotchets, quavers, semiquavers and triplets - but I struggle working out the count when a bar starts with a quaver, crotchet, quaver, crotchet, crotchet. Can you tell me if there is some sort of system that I can apply to counting rhythms?

Special K

The answer:

It doesn't matter if you're dealing with a simple rhythm or more complex ones, the answer is always the same: learn to count through them. The fundamentals don't really change that much from one to the other, it's really down to the successful application of counting regimes.

So let's examine the rulebook for decoding the dots. As with everything, it's best to start at a pretty basic level and work upwards. Ex 1 looks at how we can divide a bar up mathematically.

Example 1

We start with a whole note (or semibreve), then divide it in two and end up with two half notes (or minims), halve things again and get four quarter notes (or crotchets). Apply the same logic once more and we find the bar has now been divided into first eight equal parts (eighth notes or quavers) and finally into sixteenths (or semiquavers).

Dividing again will give us the smaller elements in music's rhythmic currency like thirty-second notes (demisemiquavers) etc; what we have in Ex 1 are arguably the most common 'domestic' rhythmic elements. Here (and we really can't stress this enough) you've got to be really sure-footed when it comes to counting. These might be the basics but, as we've seen, the idea of dividing bars up continues, theoretically speaking, in much the same way from here on.

So mastering a system of counting these beat divisions is absolutely vital to understanding what comes next. Once we're happy with how a bar may be split up like this, we can move on to look at how individual beats can also be divided. It's no surprise that the system of halving and then halving again remains in place here, too.

In Ex 2 we begin with a single beat that we then split in half (producing eighth notes) and then half again (producing sixteenths) and then once more to give us thirty-second notes.

Example 2-5

Now, what I am trying to emphasise here is that the maths is exactly the same as before. If you've grasped the fundamental idea of how a bar can be subdivided, then splitting beats shouldn't come as too much of a shock.

As an example, take a look at Ex 3: first we have a bar divided into four equal beats, producing four quarter notes, followed by a single beat split into four, producing sixteenths. It's vital here to see the similarity because, as we've already said, understanding things at this level will aid you later on. Obviously, there comes a time when things are slightly less straightforward - after all, if music was so neatly split into symmetrical patterns like this it would be very boring indeed.

Ex 4 illustrates one way we can throw a curve into the proceedings and split a bar into four groups of three - in other words, triplets. Once again, this is a very common way of going about things, and it's possible to split beats into five, seven, nine or practically anywhere your musical imagination wants to take you.

However, we maintain that if you can cope with counting triplets, the other denominations shouldn't necessarily prove too much of a conceptual strain when you get to meet them. You might think that once we introduce rests into counting, things are set to become more complex.

But why? A rest is just a beat (or part thereof) that you don't play - counting it is exactly the same. Look at Ex 5 and you'll see what we mean. Here's a simple example: a bar of four, followed by another with beats two and four rested. But the counting procedure is identical in both cases. You simply don't play anything where directed in the second bar, but you would count through it the same way.

If we do this we count the notes you play out loud and the ones you don't silently in your head, if that helps. Dots also tend to phase people, but as long as your understanding of counting is solid, they shouldn't be too much of a bother. In effect, a dot after a note means that it lasts for one and a half times its value. So a quarter note would last for one and a half beats - and so on.

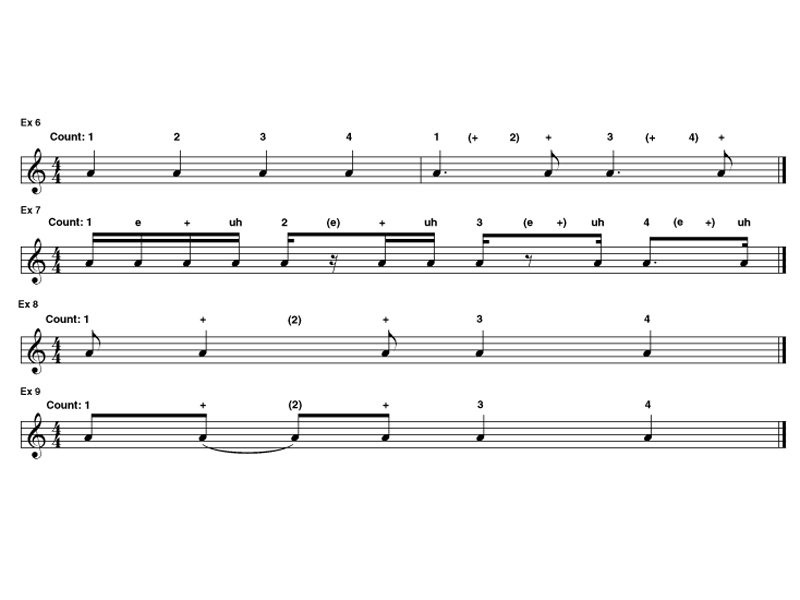
Example 6-9

Look at Ex 6 - here we have a bar of four followed by another bar containing some dotted notes. The trick here (if there is one) is to reduce everything to its smallest part when you count. So if we count the second bar in eighths, there shouldn't be a problem and the eighth notes at the end of the second and fourth beats should be rhythmically spot on.

Remembering that anything you can do to a bar you can do to a beat, it's pretty much a normal day at the office for Ex 7. The first beat is split evenly into four sixteenths whereas the second, third and fourth are either rested or dotted. But in all cases, you count them the same way. The only trick is to be able to tell one from the other - played or rested, played or dotted.

We can't go much further into the realms of written music here, but you should see that there is some consistency involved in rhythmic notation. Music is renowned for having more than one way of writing the same thing down.

In the latter part of your question you've stumbled on what we call the 'American system' of writing rhythm. We were always taught that it was musical etiquette to keep the individual beats clear in any piece of music - and it's certainly the way we transcribe even today.

In the American system you get groupings like the one you describe (see Ex 8). Here, a quarter note is effectively bridging the gap between beats one and two and you would count it the way we have written over the top. However, if we were writing this exact same rhythm down, we would write it like Ex 9.

As you can see, the counting is exactly the same in essence - and, of course, so is the rhythm - but the argument is that Ex 8 is easier to read. The jury is still out on this one so, consequently, both methods are in use and today's music reader has to be prepared to meet both out there in the field. But to our minds, we still think that Ex 9 is the easier of the two to read as it clearly defines the original beats.

Summary

So, to sum up, we think it's a case of going back to the drawing board for a while and spending some time shoring up the foundations of your rhythm reading. Spend a little time every day just taking a few bars of music and working out a counting regime for them.

If you use the music in GT for this, you can always check your results with the CD. You'll be surprised at how soon it begins to look more logical and user-friendly, and pretty soon you probably won't even have to think about it any more.